Principal Moment of Inertia: Asymmetric Cross-section (Part 2)

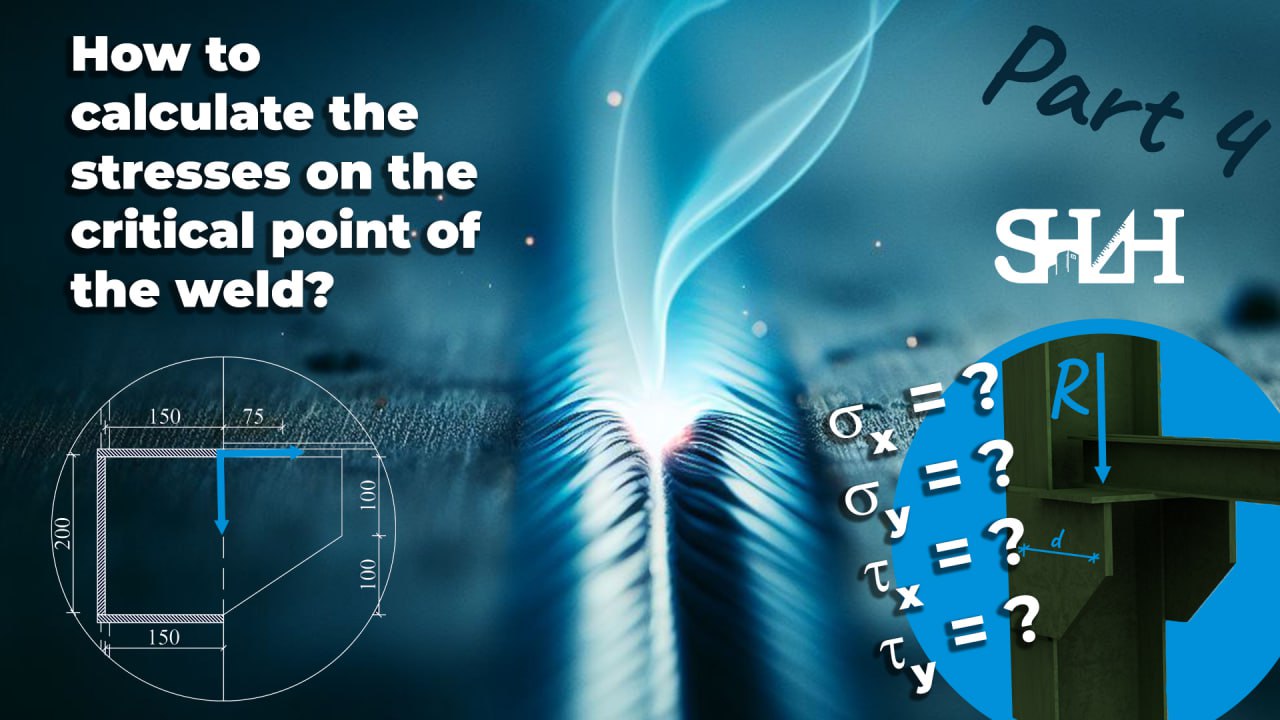
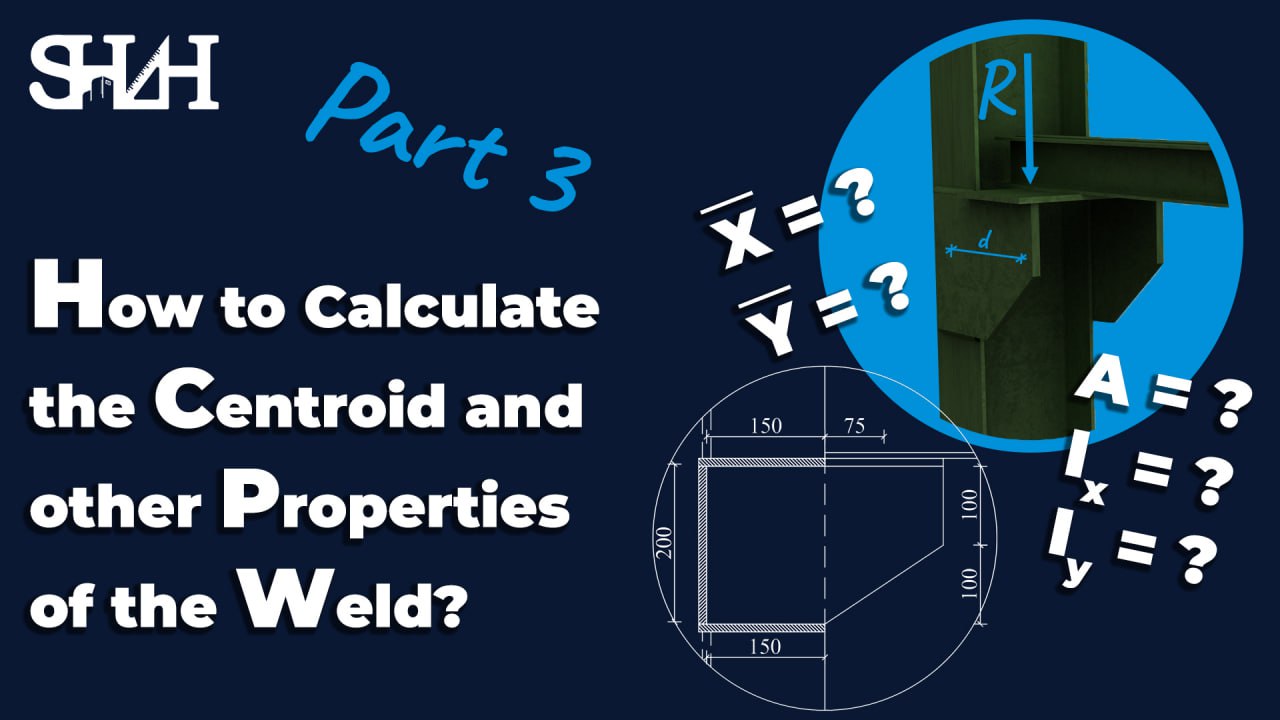
In the previous video, we covered the calculation of two important properties of an asymmetric cross-section – the centroid and the moment of inertia. Specifically, we determined the location of the centroid and evaluated the moment of inertia about two axes – one horizontal and the other vertical – that pass through the centroid. In this video, we will be building upon the knowledge gained in the previous video and delving deeper into the topic by using Mohr’s circle. Specifically, we will leverage Mohr’s circle to calculate two important parameters – the principal axes and the angle between the principal axes and the two horizontal and vertical axes passing through the centroid. The principal axes are the two orthogonal axes about which the moment of inertia is maximum and minimum, respectively. Knowing the location of these principal axes is crucial in understanding the behavior of the cross-section under different loading conditions. Additionally, the angle between the principal axes and the horizontal and vertical axes passing through the centroid is an important parameter that can be used to determine the orientation of the cross-section with respect to the loading direction. By the end of this video, you will have a clear understanding of how Mohr’s circle can be used to calculate the principal axes, the angle between these axes and the horizontal and vertical axes passing through the centroid of an asymmetric cross-section.

Link to the description and detailed solution notes: Download